In this instance, we will investigate and resolve the remarkable quadratic equation 4x ^ 2 – 5x – 12 = 0. The quadratic equation is now a crucial component of every institution’s mathematics curriculum, including colleges and universities.
All things have vital applications in many other disciplines of study that are more focused on research and development, thus while many people may believe that quadratic has no practical usefulness, this is a misunderstanding.
Quadratic Equation
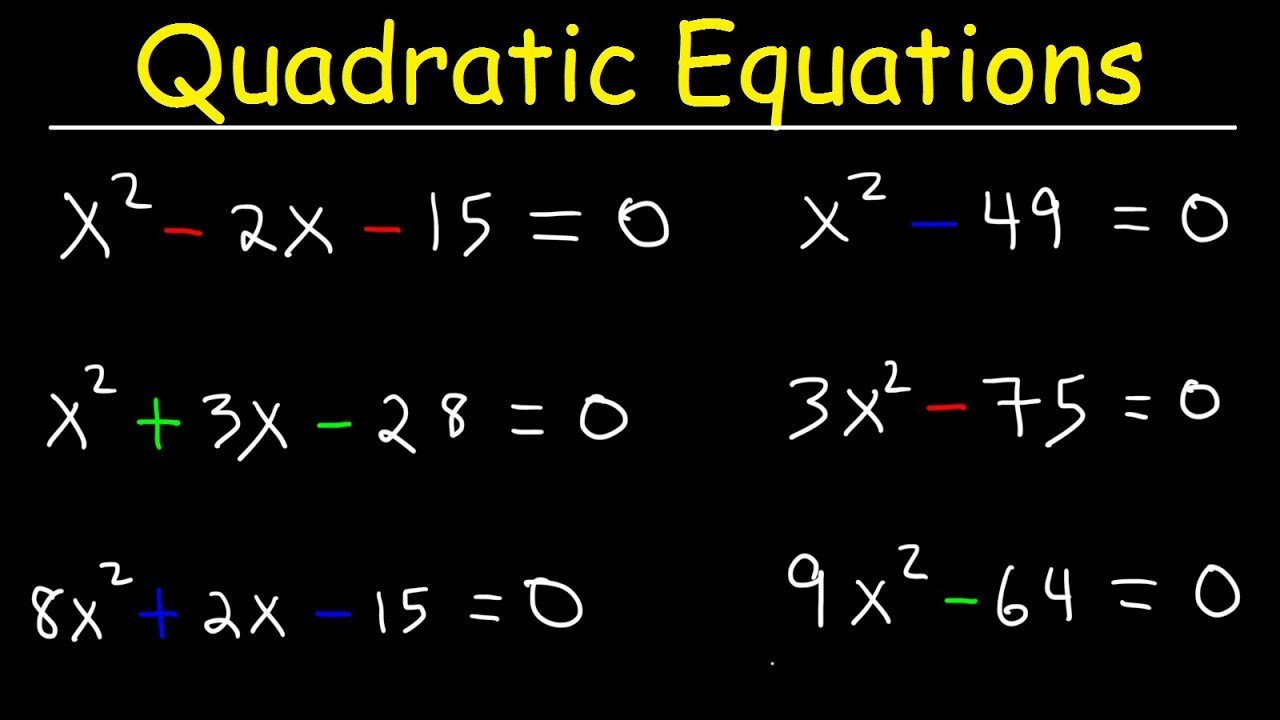
The quadratic equation is one of the most significant concepts in algebra. Without knowing this, one may lose out on a crucial component of learning about a significant area of mathematics. This algebraic category is regarded as one of the essential ideas. We will examine quadratic equations in more detail in our post today, and we have included a thorough solution to 4x ^ 2 – 5x – 12 = 0.
Concept of Quadratic Equation
A quadratic equation is a second-degree polynomial equation, which simply indicates that the equation has at least one squared element. This is how the quadratic equation is expressed in general form. Ax2 + Bx + C = 0
where the variable is represented by x and the constants are a, b, and c. This article will answer an equation for us, which we will set aside afterward. The equation that has to be solved is that.
Understanding the Equation 4x ^ 2 – 5x – 12 = 0
Quadratic equation formulae may be used to solve this problem with ease. The direct technique and the Sridhar Acharya method are the two approaches available for solving this problem. But it’s often preferable to just use the direct way because of this equation.
Process to Solve the Quadratic Equation

The steps listed below must be followed to solve the quadratic equation:
-
Solve using the Factoring Method:
The quadratic equation may be expressed as the sum of two binomials using the factoring method. In situations when the problem is easily factorable, this is a very successful strategy. If we set all of the binomials’ roots to 0, we can find the roots. We can then ascertain what x may be.
-
Solve using the Quadratic Formula:
We may also utilize the universal formula to solve the above equation. If we replace a, b, and c from our equation with x = (-b ± √(b^2 – 4ac)) / 2a, we get 4x ^ 2 – 5x – 12 = 0.
-
Solving By Completing the Square
This is yet another fantastic method for resolving the quadratic issue. By transforming the provided equation into a trinomial square, this method simplifies the process of solving the roots.
Root Patterns with Discriminant Methods
When understanding the nature of roots for any quadratic equation, this discriminant is a helpful category. The roots of the formula b^2 – 4ac may be real and various, real and similar, or distinct based on the discriminant value.
Every stage is described below:
-
Getting Real and Diverse Roots
Two different variable roots of the quadratic equation are obtained when the discriminant value is more than zero. It indicates where the equation crosses the x-axis in a graphical representation.
-
Getting Real and Similar Roots
The equation arrives at a common x-axis point using the same graphical method. It happens when the discriminant value is 0.
Uses Of Quadratic Equation
Although quadratic equations might not seem like they are particularly useful in today’s society, they are quite significant for several additional reasons. The following is a list of situations in which quadratic equations are very helpful:
- Physics is among the fields that perform complex computations using quadratic equations. Quadratic equations are used to compute projectile motion equations and other fundamental physics principles.
- Engineering uses quadratic equations a lot to solve equations. These formulae might be related to signal processing, structural analysis, or electrical circuits.
- Contrary to popular belief, quadratic equations are utilized in the study of finance, especially when simulating complex financial systems and determining tax investment returns.
Conclusion
A good example of a second-degree polynomial equation is the quadratic equation 4x ^ 2 – 5x – 12 = 0. This blog post explains every important step that can help to simplify the issue and make the solution more accessible. It has also been argued how useful the quadratic equation is in contemporary life. Since more investigation will uncover many more characteristics and applications for quadratic equations than just this one.
FAQs
What does the root of a quadratic equation mean?
The calculated value of x is referred to as the quadratic equation’s roots, and it may be obtained using the factoring technique and the quadratic formula, both of which are discussed in this article.
Are there any complex roots for quadratic equations?
In reality, complicated roots may occur if the discriminant’s estimated value is smaller than 0 and in a negative term.
Are there any practical uses for quadratic equations?
It plays a significant part in several areas of our daily life and is necessary for many others, including the study of physics, engineering, design, finance, economics, and other topics.
How can we better understand quadratic equations?
Many websites offer helpful solutions for handling any kind of quadratic problem. Popular choices include math textbooks, instructional websites, and math-related YouTube channels.
Read More: SAHARA REFUND || INFO & DETAILS
